Magnetic Force on a Current Carrying Wire

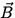Let us consider a long straight wire carrying a current $\vec{I}$ in a magnetic field $\vec{B}$ . Each charge q in the wire will experience a force, and it is possible to find the total force on the wire by the following arguments. Suppose there are n charges per unit volume in the wire of cross-sectional area A and length l , as in Fig. 1.4.

  

Figure 1.4: Current carrying wire in a magnetic field

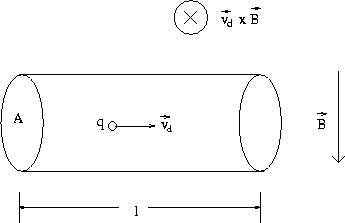If each charge has a charge q and is moving with a drift velocity $\vec{v}_{d}^{}$, then there will be a total force on the wire given by

 

$$\vec{F} \vec{v}_{d}^{} \vec{B} \vec{I} \vec{B}$$ (4)

where we recall that $\vec{I}$ = nq$\vec{v_d}$A and also that by convention $\vec{I}$ is in the direction of the flow of positive charges.