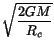 = c
= c
Einstein's special theory of relativity states, however, that nothing can travel faster than the speed of light, which is:
According to the above argument we should be able to form black holes simply by compactifying any mass to a small enough radius, Rc. We can calculate what this critical radius is for any given mass using our previously derived expression for escape velocity. In particular, the critical radius is the value of R for which ve = c. Thus, we have:
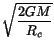 = c
= c
Black holes are therefore regions of space that are so densely packed with matter that nothing, not even light can escape. More specifically, the escape velocity of matter with the region bounded by the black hole is greater than the speed of light. Once you fall inside this region, it is impossible to escape, and no one outside this region can observe anything that is going on inside. For this reason, the boundary between the interior and exterior of a black hole, the ``surface of no return'' is called an event horizon.
At the center of a black hole is a ``singularity'', where all the mass of the black hole is concentrated and the known laws of physics break down. The density of matter at the singularity is effectively infinite. Once you fall below the event horizon of a black hole you are irrevocably sucked into the singularity at the center. Time and space literally change roles inside a black hole and you can no more avoid moving towards the center than you can avoid moving forward in time from 2:00pm to 3:00pm.
Black holes cannot be created in a laboratory. The concentration of matter to make a reasonable sized black hole is too large. For example to form a black hole the size of a baseball, you would have to pack the entire mass of the Earth into a region about 5 cm across. Black holes can form form astronomical objects by gravitational collapse. If a star uses up its thermonuclear fuel, there is no longer any pressure keeping the gas in the star from collapsing under its own weight, and the star collapses. If the star's mass is more than about 2-3 times that of our sun, no known force can prevent it from collapsing into a black hole. Such a black hole would be about 5-10 km in size. There is strong observational evidence for the existence of black holes in binary star systems (i.e. black holes orbiting visible stars) and at the center of most galaxies. One such black hole, at the center of galaxy M87, is thought to contain the mass of 5 billion suns.